Fastest Addition and Subtraction techniques - Math Tricks

SIMULTANEOUS ADDITION AND SUBTRACTION

In Mathematics Addition, Subtraction, Multiplication and Division are four basic operations of Arithmetic. But how to add numbers quickly and easily? In this post i will explain one of the best method for adding and subtracting large numbers easily in a single line within mind. Faster operations of 'BODMAS' can save our time a lot during solving mathematical problems.

There are 3 types of Cases

 CASE 1:  All digits of answer are positive:
Example 1: 432 - 83 + 71 = ?
Solution: For unit digit of our answer, add and subtract the digits at units places according to the sign attached with the respective numbers. Here unit digit of our temporary result is: 2 - 3 + 1 = 0
Write it like this: 432 - 83 + 71 = _ _ (0)

Now add and subtract the digits at tens place: 3 - 8 + 7 = 2
We can write it as: 432 - 83 + 71 = _ (2) (0)

Similarly temporary value at hundreds place will be: 4
So we can write it as: 432 - 83 + 71 = (4) (2) (0)

Answer will be 420.

 CASE 2:   Few digits are negative but leftmost positive:
Example 2: 522 - 304 + 61 = ?
Solution:  Step 1:  For unit digit of our answer, add and subtract the digits at units places according to the sign attached with the respective numbers. In this example the unit digit of our temporary result is: 2 - 4 + 1 = (-1)
We can write as: 522 - 304 + 61 = _ _ (-1)

Now add and subtract the digits at tens place: 2 - 0 + 6 = 8
We can write as: 522 - 304 + 61 = _ (8) (-1)

Similarly temporary value at hundreds place will be: 5 - 3 = 2
We can write as: 522 - 304 + 61 = (2) (8) (-1)

 Step 2:  Now above figures have to be changed into real value:
To replace -1 by a positive digit we borrow from digits at tens or hundreds place.
We borrow 1 from tens which becomes 10 at units place, leaving (8 - 1 = 7) at tens place.
Thus at units place (10 - 1 = 9)

So our final result will be = 279

We can also write (2) (8) (-1) = 280 - 1 = 279

Example 3: 6224 - 939 + 642 - 345 =?
Solution :  Step 1:  Temporary figures are:
6224 - 939 + 642 - 345 = (6) (-4) (-1) (-8)

 Step 2:  Now above figures have to be changed into real value:
To replace -8 by a positive digit we borrow from digits at tens place.
We borrow 1 from tens place which becomes 10 at units place, leaving (-1 -1 = -2) at tens place. Thus at units place (10 - 8 = 2)

We borrow 1 from hundreds place which becomes 10 at tens place, leaving (-4 -1 = -5) at hundreds place. Thus at tens place (10 - 2 = 8) and at hundreds place (10 - 5 = 5)

Required answer will be: 5582

NOTE: After step 1 we can also write like this: 6000 - 418 = 5582
But this method can't be combined with step 1 to perform simultaneously in a single line. So, we should try to combine step 1 with step 2.

Example 4: 73216 - 8396 + 3510 - 999 =?
Solution :  Step 1:  Temporary figures are:
73216 - 8396 + 3510 - 999 = (7) (-2) (-5) (-16) (-9)

 Step 2:  Now above figures have to be changed into real value:
Units digit = 10 - 9 = 1 [1 borrowed from (-16) result (-16 - 1 = -17)]
Tens digit = 20 - 17 = 3 [2 borrowed from (-5) result (-5 - 2 = -7)]
Hundreds digit = 10 - 7 = 3 [1 borrowed from (-2) result (-2 - 1 = -3)]
Thousands digit = 10 - 3 = 7 [1 borrowed from (7) result (7 - 1 = 6)]
Required answer = 67331  

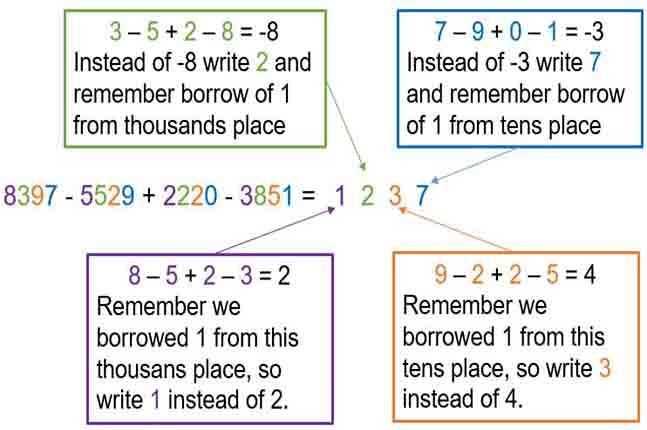
Example 5: 8397-5529+2220-3851 = ?
Solution:
 Step 1:  (2) (-8) (4) (-3)
 Step 2:  (1)  (2) (3)  (7)

Now combine both steps 1 and 2 and try to solve it in a single line:

 CASE 3:  Leftmost digit of answer is negative:
In this case, subtract the rightmost digit from 10 and rest from 9. Also leftmost digit will be decreased by 1.

Example 6: 6248 - 8524 + 2637 - 6182 = ?
Solution:  Step 1:  (-6) (1) (7) (9)
Since the leftmost digit is negative, we need to change the digits:
9 will become 1(=10 - 9) , 7 will become 2(=9 - 7) and 1 will become 8(=9 - 1). Instead of -6 we will write -5. So final answer will be = -5821

We can also write it as: -6000 + 179 = -5821 but there is no need to write. Just try to solve in mind.

Checking the Calculation
Digit-Sum Method: This method is known as Nines-Remainder method.

• We get the digit sum of a number by "adding across" the number. For example: the digit sum of 13011 is 6 (= 1 + 3 + 0 + 1 + 1).

• We always reduce the digit sum to a single figure if it is not already a single figure. For example: the digit sum of 35684 is = 3 + 5 + 6 + 8 + 4 = 26 = 2 + 6 = 8

• In "adding across" a number, we may drop out 9's. Thus, if we happen to notice two digits that add up to 9, such as 2 and 7, we ignore both of them; so the digit sum of 90723 is 3 at a glance.

• Because 'nines don't count' in this process, a digit sum of 9 is the same as a digit sum of zero. For example: The digit sum of 441 is zero.

Basic Rule: Whatever we do to the numbers, we also do to their digit-sum; then the result that we get from the digit-sum of the numbers must be equal to the digit-sum of the answer.

For example: In example 1:  432 - 83 + 71 = 420
Taking left hand side = (4 + 3 + 2) - (8 + 3) + (7 + 1) = 9 - 2 +8 = 6
Taking right hand side = 4 + 2 + 0 = 6
Digit sum of numbers = Digit sum of the answer, so calculation is correct.

You don't need to write step 1, 2, 3 etc., you need to solve such addition and subtraction in a single line within mind. It may look lengthy but it is the shortest method of addition. Also method of checking the calculation is also very easy. You need to practice two to three times only. Good Luck 👍